What’s a logical paradox?
Preface
 This was written in 2009. (Revised 2022.) It explains the notion of a logical paradox in a way that comes naturally to me. The conclusion drawn at the end is probably the main thing for me (“We are blinder than we can imagine in matters of straightforward logical reasoning”) but it is not always emphasized by others!
This was written in 2009. (Revised 2022.) It explains the notion of a logical paradox in a way that comes naturally to me. The conclusion drawn at the end is probably the main thing for me (“We are blinder than we can imagine in matters of straightforward logical reasoning”) but it is not always emphasized by others!
 This was written in 2009. (Revised 2022.) It explains the notion of a logical paradox in a way that comes naturally to me. The conclusion drawn at the end is probably the main thing for me (“We are blinder than we can imagine in matters of straightforward logical reasoning”) but it is not always emphasized by others!
This was written in 2009. (Revised 2022.) It explains the notion of a logical paradox in a way that comes naturally to me. The conclusion drawn at the end is probably the main thing for me (“We are blinder than we can imagine in matters of straightforward logical reasoning”) but it is not always emphasized by others!
7. The heap
Consider the myriad coloured squares below and these two obvious facts about them:
These facts may seem innocuous but they in fact generate a paradox since they allow us to “deduce” that the last square looks red, which is patently false, as the last square is clearly blue.
All the same, the “deduction” is as follows:
What is clear is that the squares change their colour ever so gradually—imperceptibly—from one square to the next. This explains how you can start with a red square and end up eventually with a blue one. Put another way, numerous imperceptible differences can add up to a perceptible one, so that, even if two adjacent squares always look the same, two distant squares in the series may not do so.
Some people think that pointing this out “resolves” the paradox, but it’s not that simple, of course, because it does not tell us where the reasoning above goes wrong, which is the crucial thing. After all, the two statements that we began with remain true:
The ancient Greeks themselves discussed the paradox in terms of a heap of sand, or sometimes wheat.
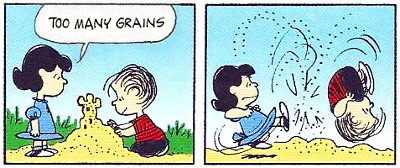
Consider a heap of sand—comprised of a billion grains, say, which is roughly the amount pictured below. If you remove just one grain of sand from this heap, then what remains, the Greeks observed, will still be a heap of sand. After all, a single grain of sand can surely make no difference to whether or not the accumulation before you is a “heap” of sand. (Can it?)
will still be a heap of sand. After all, a single grain of sand can surely make no difference to whether or not the accumulation before you is a “heap” of sand. (Can it?)
Repeated reasoning then “proves” that, no matter how many grains of sand you remove, you will still have a heap of sand, even if you pare it down to just one grain! The reasoning, as before, is this:
Amazingly, there is no consensus to this day on exactly where the reasoning above goes wrong, although various ideas have been proposed. Let’s examine a couple of them here, which we may discuss in terms of the heap of sand. (Similar things may be said of the coloured squares.)
 Some people trace the problem to the term ‘heap,’ which they accuse of being vague and not precisely defined. In particular, we are not told exactly how many grains of sand count as a “heap.” As soon as we have a precise definition, they say—e.g., that 10,000 grains (or more) count—it will be evident that removing one grain can make a difference to whether or not an accumulation of grains before you is a heap. By definition, it will make a difference when you have 10,000 grains!
Some people trace the problem to the term ‘heap,’ which they accuse of being vague and not precisely defined. In particular, we are not told exactly how many grains of sand count as a “heap.” As soon as we have a precise definition, they say—e.g., that 10,000 grains (or more) count—it will be evident that removing one grain can make a difference to whether or not an accumulation of grains before you is a heap. By definition, it will make a difference when you have 10,000 grains!
This way of “rejecting the reasoning,” favoured by the American logician and philosopher W. V. Quine (pictured above), among others, assumes that such vagueness or imprecision is a defect of language that stands to be rectified. “Always make your terms precise,” they say. This may seem sensible at first glance, but, as Quine himself acknowledges, it does run into the fact that ordinary language is chock full of vague terms like ‘heap,’ ‘bald,’ ‘tall,’ ‘dark,’ ‘fast,’ ‘cloud,’ ‘hill,’ ‘adult,’ ‘baby’—the list is endless. Not only is it practically impossible to make all of these terms precise, it is unclear if this is even desirable.
For example, in the majority of contexts in which the term ‘heap’ is used, a single grain really makes no difference to what counts as a heap. If you are building a sandcastle, say, and need a heap of sand, it would make no difference if your “heap” contained a grain more, or less, of sand. One grain would make no difference.
If you require a heap of sand for building a road, then your heap would need to be larger, of course, but, even so, one grain of sand would make no difference. In cases like these, a “heap” of sand is just whatever (bell-shaped) accumulation of sand befits the purpose at hand, the precise number of grains being immaterial.
And even if there was some special context where the precise number of grains did matter, there are plenty of others where it doesn’t. So it seems misguided to want always to replace a vague term with a precise one: sometimes the vagueness serves a purpose. From this point of view, the flaw in the reasoning above cannot really be blamed on the vagueness of the term ‘heap,’ since the word is supposed to be vague. Nor, in tandem, can it be blamed on the premise that one grain of sand can never make a difference between a heap and a non-heap. As the term ‘heap’ is typically used, this premise is essentially true!
 Moved by these concerns, some people have suggested a completely different way of “rejecting the reasoning.”
Moved by these concerns, some people have suggested a completely different way of “rejecting the reasoning.”
Granting that words like ‘heap’ and ‘red’ are meant to be vague, they have attempted instead to formulate a more adequate logic for them. Classical logic, they say, is too rigid to handle vague terms, particularly in its insistence that any given accumulation of grains must be either a heap or else not a heap, as though this was always a black and white affair. Why not admit degrees of heaphood instead?, they suggest.
On this view, championed by the American philosopher Kenton Machina (pictured above), among many others, the fewer the number of grains in a given accumulation, the less of a heap it will be, and vice versa. So if you start with a billion grains and keep removing grains, there will be a gradual “dwindling” of heaphood as the number of grains abates. Along the way, there won’t always be a definitive (yes or no) answer as to whether a heap stands before you, but perhaps only a greater or lesser degree of truth to the matter.
By these lights, the mistake in the reasoning was to fail to notice this gradual dwindling (or leakage) of truth. Consider, for example, our opening syllogism:
owing to the slight dwindling of heaphood caused by the removal of one grain. So the conclusion, when drawn, ends up being slightly less true than the first premise.
So the syllogism is not watertight, where truth is concerned, and, by the time we are down to a single grain, the conclusion will have been completely drained of truth. (“A single grain is definitely not a heap.”) This account is held to capture the “logic” of vague terms better.
As promising as this account may seem, it faces its own hurdles. Many of these are technical ones, relating to the idea that truth comes in degrees. Thus, what determines the degree of truth of a given statement? What determines how much truth is transmitted across a logical inference from premises to conclusion? And so on. These difficulties are vexed and we can’t examine them here, but here’s a simple example of one such hurdle, just to give the idea.
Suppose that a billion grains is definitely a heap—i.e., possesses the maximum degree of heaphood. At which point, as we keep removing grains from it, will the remaining grains cease to be definitely a heap?—At how many grains? If you think about this for a while, you may realize that the question is impossible to answer, because ‘definitely a heap’ seems to be just as vague as ‘heap’! But this means that we can regenerate the whole paradox using ‘definitely a heap’ instead:
 To be fair, all of these matters are still up in the air and consensus on them remains elusive. We’ve only scratched the surface thus far, but you can find out more about the above two and various other attempts to resolve this ancient paradox from this book (shown on the right) or from this Stanford encyclopedia entry.
To be fair, all of these matters are still up in the air and consensus on them remains elusive. We’ve only scratched the surface thus far, but you can find out more about the above two and various other attempts to resolve this ancient paradox from this book (shown on the right) or from this Stanford encyclopedia entry.
For the moment, let’s leave the paradox unresolved and return briefly to the point made in the previous section. Recall our observation that a line of reasoning may have a flaw so subtle that we’d never suspect its existence but for the fact that it culminates in a crazy conclusion. The paradox of the heap illustrates this well since it is only the outrageous conclusion (“One grain of sand is a heap”) that makes us question the reasoning. If the conclusion had seemed banal, or otherwise unremarkable, we would almost certainly have bought the reasoning! The proof of this is that people unfamiliar with this paradox employ this sort of reasoning all the time, perfectly convinced of their logic!
For example, opponents of abortion often point out that if it is impermissible to abort a foetus one hundred days after conception, when the foetus is recognizably human, then it must be impermissible ninety-nine days after conception too.
After all, one day can’t make a difference – the foetus doesn’t suddenly turn human overnight!
Repeated reasoning then “proves” that the foetus cannot permissibly be aborted even one day after conception, notwithstanding its then just being a clump of cells.
We cannot blame them for seeing no flaw in this logic, since even those who know that there is a flaw cannot agree on what it is.
This sort of case should worry us, for how many others like it exist that we have no inkling of? In the present case, we are lucky enough to have realized that the given style of reasoning is flawed, even if we can’t quite pin down the flaw, and so word can at least go around not to trust this style of reasoning. (It is one version of the so-called slippery slope fallacy.)
But might there be other cases where it hasn’t yet dawned upon us that a certain style of reasoning is flawed, even though we employ it all the time, perfectly convinced of its validity? The answer is almost certainly yes, so the only question is how widespread the phenomenon is.
Unfortunately, by its very nature, we have no real way of knowing. But there is reason to suspect that the phenomenon is more widespread than we might think. Let’s consider another famous paradox where the same danger proves to arise.
Consider the myriad coloured squares below and these two obvious facts about them:
The first square looks red.
Colourwise, any two adjacent squares look the same.
Colourwise, any two adjacent squares look the same.
These facts may seem innocuous but they in fact generate a paradox since they allow us to “deduce” that the last square looks red, which is patently false, as the last square is clearly blue.
All the same, the “deduction” is as follows:
The first square looks red.
But any two adjacent squares look the same.
Ergo, the second square looks red.
But any two adjacent squares look the same.
Ergo, the third square looks red.
The reasoning is brutally simple, but it must surely contain a flaw, since the bottom line (“The last square looks red”) cannot possibly be swallowed. This was known to the ancient Greeks, who knew this paradox well, but, to this day, despite no shortage of suggestions, it remains unclear where exactly the reasoning goes wrong.But any two adjacent squares look the same.
Ergo, the second square looks red.
But any two adjacent squares look the same.
Ergo, the third square looks red.
⋮
Ergo, the last square looks red.What is clear is that the squares change their colour ever so gradually—imperceptibly—from one square to the next. This explains how you can start with a red square and end up eventually with a blue one. Put another way, numerous imperceptible differences can add up to a perceptible one, so that, even if two adjacent squares always look the same, two distant squares in the series may not do so.
Some people think that pointing this out “resolves” the paradox, but it’s not that simple, of course, because it does not tell us where the reasoning above goes wrong, which is the crucial thing. After all, the two statements that we began with remain true:
The first square looks red.
Colourwise, any two adjacent squares look the same.
But, from these two statements, we seem able to “prove” that the last square looks red, as demonstrated above. This reasoning needs to be addressed explicitly, which is what has proven difficult to do.Colourwise, any two adjacent squares look the same.
The ancient Greeks themselves discussed the paradox in terms of a heap of sand, or sometimes wheat.
Consider a heap of sand—comprised of a billion grains, say, which is roughly the amount pictured below. If you remove just one grain of sand from this heap, then what remains, the Greeks observed,

Repeated reasoning then “proves” that, no matter how many grains of sand you remove, you will still have a heap of sand, even if you pare it down to just one grain! The reasoning, as before, is this:
A billion grains is a heap.
The conclusion of this reasoning (“One grain is a heap”) is again absurd and virtually everyone agrees that it cannot be swallowed. So, as with the coloured squares, it seems that we must “reject the reasoning”—the only question being how. Following the Greeks, this is often called the paradox of the heap. It is one of the oldest, simplest, and yet hardest paradoxes around. Removing one grain from a heap still leaves you with a heap.
Ergo, 999,999,999 grains is a heap. Removing one grain from a heap still leaves you with a heap.
Ergo, 999,999,998 grains is a heap⋮
Ergo, one grain is a heap.Amazingly, there is no consensus to this day on exactly where the reasoning above goes wrong, although various ideas have been proposed. Let’s examine a couple of them here, which we may discuss in terms of the heap of sand. (Similar things may be said of the coloured squares.)
⚹

This way of “rejecting the reasoning,” favoured by the American logician and philosopher W. V. Quine (pictured above), among others, assumes that such vagueness or imprecision is a defect of language that stands to be rectified. “Always make your terms precise,” they say. This may seem sensible at first glance, but, as Quine himself acknowledges, it does run into the fact that ordinary language is chock full of vague terms like ‘heap,’ ‘bald,’ ‘tall,’ ‘dark,’ ‘fast,’ ‘cloud,’ ‘hill,’ ‘adult,’ ‘baby’—the list is endless. Not only is it practically impossible to make all of these terms precise, it is unclear if this is even desirable.
For example, in the majority of contexts in which the term ‘heap’ is used, a single grain really makes no difference to what counts as a heap. If you are building a sandcastle, say, and need a heap of sand, it would make no difference if your “heap” contained a grain more, or less, of sand. One grain would make no difference.
If you require a heap of sand for building a road, then your heap would need to be larger, of course, but, even so, one grain of sand would make no difference. In cases like these, a “heap” of sand is just whatever (bell-shaped) accumulation of sand befits the purpose at hand, the precise number of grains being immaterial.
And even if there was some special context where the precise number of grains did matter, there are plenty of others where it doesn’t. So it seems misguided to want always to replace a vague term with a precise one: sometimes the vagueness serves a purpose. From this point of view, the flaw in the reasoning above cannot really be blamed on the vagueness of the term ‘heap,’ since the word is supposed to be vague. Nor, in tandem, can it be blamed on the premise that one grain of sand can never make a difference between a heap and a non-heap. As the term ‘heap’ is typically used, this premise is essentially true!
⚹

Granting that words like ‘heap’ and ‘red’ are meant to be vague, they have attempted instead to formulate a more adequate logic for them. Classical logic, they say, is too rigid to handle vague terms, particularly in its insistence that any given accumulation of grains must be either a heap or else not a heap, as though this was always a black and white affair. Why not admit degrees of heaphood instead?, they suggest.
On this view, championed by the American philosopher Kenton Machina (pictured above), among many others, the fewer the number of grains in a given accumulation, the less of a heap it will be, and vice versa. So if you start with a billion grains and keep removing grains, there will be a gradual “dwindling” of heaphood as the number of grains abates. Along the way, there won’t always be a definitive (yes or no) answer as to whether a heap stands before you, but perhaps only a greater or lesser degree of truth to the matter.
By these lights, the mistake in the reasoning was to fail to notice this gradual dwindling (or leakage) of truth. Consider, for example, our opening syllogism:
A billion grains is a heap
On the classical view, since both premises are true, the conclusion must be true as well, which begets the slippery slope. On the current view, however, truth comes in degrees. The first premise possesses the maximum degree of truth, let us suppose. (“A billion grains is definitely a heap.”) And the second premise has a high degree of truth, though not the maximum one, Removing one grain from a heap still leaves you with a heap
Ergo, 999,999,999 grains is a heapowing to the slight dwindling of heaphood caused by the removal of one grain. So the conclusion, when drawn, ends up being slightly less true than the first premise.
So the syllogism is not watertight, where truth is concerned, and, by the time we are down to a single grain, the conclusion will have been completely drained of truth. (“A single grain is definitely not a heap.”) This account is held to capture the “logic” of vague terms better.
As promising as this account may seem, it faces its own hurdles. Many of these are technical ones, relating to the idea that truth comes in degrees. Thus, what determines the degree of truth of a given statement? What determines how much truth is transmitted across a logical inference from premises to conclusion? And so on. These difficulties are vexed and we can’t examine them here, but here’s a simple example of one such hurdle, just to give the idea.
Suppose that a billion grains is definitely a heap—i.e., possesses the maximum degree of heaphood. At which point, as we keep removing grains from it, will the remaining grains cease to be definitely a heap?—At how many grains? If you think about this for a while, you may realize that the question is impossible to answer, because ‘definitely a heap’ seems to be just as vague as ‘heap’! But this means that we can regenerate the whole paradox using ‘definitely a heap’ instead:
A billion grains is definitely a heap;
which would appear to defeat the point of introducing degrees of heaphood in the first place. Removing one grain from something that is definitely a heap still leaves you with something that is definitely a heap.
Ergo, 999,999,999 grains is definitely a heap;⋮
Ergo, one grain is definitely a heap
⚹
For the moment, let’s leave the paradox unresolved and return briefly to the point made in the previous section. Recall our observation that a line of reasoning may have a flaw so subtle that we’d never suspect its existence but for the fact that it culminates in a crazy conclusion. The paradox of the heap illustrates this well since it is only the outrageous conclusion (“One grain of sand is a heap”) that makes us question the reasoning. If the conclusion had seemed banal, or otherwise unremarkable, we would almost certainly have bought the reasoning! The proof of this is that people unfamiliar with this paradox employ this sort of reasoning all the time, perfectly convinced of their logic!
For example, opponents of abortion often point out that if it is impermissible to abort a foetus one hundred days after conception, when the foetus is recognizably human, then it must be impermissible ninety-nine days after conception too.
After all, one day can’t make a difference – the foetus doesn’t suddenly turn human overnight!

Repeated reasoning then “proves” that the foetus cannot permissibly be aborted even one day after conception, notwithstanding its then just being a clump of cells.
We cannot blame them for seeing no flaw in this logic, since even those who know that there is a flaw cannot agree on what it is.
This sort of case should worry us, for how many others like it exist that we have no inkling of? In the present case, we are lucky enough to have realized that the given style of reasoning is flawed, even if we can’t quite pin down the flaw, and so word can at least go around not to trust this style of reasoning. (It is one version of the so-called slippery slope fallacy.)
But might there be other cases where it hasn’t yet dawned upon us that a certain style of reasoning is flawed, even though we employ it all the time, perfectly convinced of its validity? The answer is almost certainly yes, so the only question is how widespread the phenomenon is.
Unfortunately, by its very nature, we have no real way of knowing. But there is reason to suspect that the phenomenon is more widespread than we might think. Let’s consider another famous paradox where the same danger proves to arise.
Menu
 What’s a logical paradox?
What’s a logical paradox? Achilles & the tortoise
Achilles & the tortoise The surprise exam
The surprise exam Newcomb’s problem
Newcomb’s problem Newcomb’s problem (sassy version)
Newcomb’s problem (sassy version) Seeing and being
Seeing and being Logic test!
Logic test! Philosophers say the strangest things
Philosophers say the strangest things Favourite puzzles
Favourite puzzles Books on consciousness
Books on consciousness Philosophy videos
Philosophy videos Phinteresting
Phinteresting Philosopher biographies
Philosopher biographies Philosopher birthdays
Philosopher birthdays Draft
Draftbarang 2009-2024  wayback machine
wayback machine
 wayback machine
wayback machine